"Cartesian plane" 以降の解答は各投稿の最後にあります．
直線BDは $y=\displaystyle\frac{-3}{2}(x-1)$
$=\displaystyle \frac{2}{3}R^2 h$
[Cartesian plane 解答]
(1) $y=3x$
(1) $y=3x$
(2) $x^2-y^2=1$
[Hemisphere 解答]
図の斜線部のx軸回転体になります．r-v=aとすると，
$\pi\displaystyle\int_0^a(r^2-x^2)dx=\frac{1}{3}\pi a(3r^2-a^2)$
これにr=10, a=7を代入すると，約1840[Vinculum 解答]
$\displaystyle\frac{434}{1221}$，$0.\overline{355446}$
[Shear Transformation 解答]
点Cを通りBDに平行な直線は $y-1=\displaystyle -\frac{3}{2}(x-2)$この直線と$x$軸との交点J＝$\left(\displaystyle\frac{8}{3}, 0\right)$
直線AJの傾きは -3
求める直線の$x$軸との交点をKとすると，
求める直線の$x$軸との交点をKとすると，
直線BKは $y-3=-3(x+1)$より $y=-3x$となり $K=(0, 0)$
よって求める直線AKは $y=5x$
よって求める直線AKは $y=5x$
[Closure Property 解答]
closed under multiplication and division.
not closed under addition and subtraction since 1+1=2, 1-1=0.
not closed under addition and subtraction since 1+1=2, 1-1=0.
[Duck’s Egg 解答]
(1)定義より
$\sqrt{x^2+y^2}+m\sqrt{(x-c)^2+y^2}=d$
平方根をひとつ移項して平方し，残った平方根と他方を分けてまた平方して整理すると，式(1)が導かれる．
(2)定義より
$\sqrt{(x+c)^2+y^2} \times \sqrt{(x-c)^2+y^2}=d^2$
平方して整理すると，式(2)が導かれる．
[Half-life 解答]
現在の炭素12の量からわかるこの羊皮紙の炭素14の初期値は$N_0=1.3 \times 10^{–12}$，できてからt年後に残っていた炭素14は$N=1.0 \times 10^{–12}$，$t_{1/2} = \displaystyle \frac{\ln{2}}{\lambda}$より$\lambda =\displaystyle \frac{\ln{2}}{t_{1/2}}=\frac{\ln{2}}{5730} $なので，$N=N_0 \cdot e^{-\lambda t}$より，$$1.0 \times 10^{–12} = (1.3 \times 10^{–12}) e^{-\frac{\ln2}{5730} t}$$これを解くと，$$t \approx 2170 $$この羊皮紙は2170年前のものであり，3270年前と約1100年の違いがあるので，偽造されたものと考えられる．
現在の炭素12の量からわかるこの羊皮紙の炭素14の初期値は$N_0=1.3 \times 10^{–12}$，できてからt年後に残っていた炭素14は$N=1.0 \times 10^{–12}$，$t_{1/2} = \displaystyle \frac{\ln{2}}{\lambda}$より$\lambda =\displaystyle \frac{\ln{2}}{t_{1/2}}=\frac{\ln{2}}{5730} $なので，$N=N_0 \cdot e^{-\lambda t}$より，$$1.0 \times 10^{–12} = (1.3 \times 10^{–12}) e^{-\frac{\ln2}{5730} t}$$これを解くと，$$t \approx 2170 $$この羊皮紙は2170年前のものであり，3270年前と約1100年の違いがあるので，偽造されたものと考えられる．
[Radii 解答]
(1) $S=\frac{1}{2}\cosh t \sinh t - \displaystyle \int_1^{\cosh t} \sinh t dx$
第2式$=\displaystyle \int_0^t \sinh^2 t dt=\int_0^t \frac{\cosh 2t -1}{2} dt=\left[\frac{\sinh 2t}{4}-\frac{t}{2}\right]_0^t=\frac{\sinh 2t}{4}-\frac{t}{2}$
$=\displaystyle \frac{1}{2}\cosh t \sinh t - \frac{t}{2}$
よって，$S=\displaystyle \frac{t}{2}$
(2) $V=\displaystyle \frac{1}{3}\pi r^2 (R-h) + \pi \int_{R-h}^{R} (R^2-x^2)dx$
$\displaystyle \frac{V}{\pi}=\frac{1}{3}(2Rh-h^2) (R-h) + \left[R^2 x - \frac{1}{3}x^3\right]_{R-h}^R$
$=\displaystyle \frac{1}{3}(2R^2h-3Rh^2+h^3)+ R^2 (R-(R-h)) - \frac{1}{3}(R^3-(R-h)^3)$
$=\displaystyle \frac{2}{3}R^2 h$
よって，$V=\displaystyle \frac{2}{3}\pi R^2 h$
(3) $V=\displaystyle \frac{1}{3}\pi(R^2-(R-h-b)^2)(R-h-b)+\pi\int_{R-h-b}^{R-b}(R^2-x^2) dx $
$\displaystyle - \frac{1}{3}\pi(R^2-(R-b)^2)(R-b)$
$\displaystyle \frac{V}{\pi}=$
よって，$V=\displaystyle \frac{2}{3}\pi R^2 h$
[DMS 解答]
(1) 28.2564°
(2) 36° 23’ 24”
[DST 解答]
(1) Substitute D=15 and S=6 into the formula T=D/S
T=15/6=2.5 hours
So Simon will take 2 hours and 30 minutes to cover the distance.
(2) If the D=d, take d/60 hours on going and take d/20 hours on returning. So the average speed is 2d/(d/60+d/20)=30 km/h.
[Line Graph 解答]
(1)
(2)
$\displaystyle \frac{8.4}{\sin{59°}}=\frac{9.5}{\sin{B}}$
$\sin{B}=\displaystyle \frac{\sin{59°}}{8.4}×9.5=0.9694...$
(1)
(2)
[Complete Pair & Half Pair 解答]
$\displaystyle \frac{8.4}{\sin{59°}}=\frac{9.5}{\sin{B}}$
$\sin{B}=\displaystyle \frac{\sin{59°}}{8.4}×9.5=0.9694...$
$B_1=\sin^{-1}0.9694...=75.8°$
$B_2=180-75.8=104.2$
$B=75.8°$ or $104.2°$
このように2通りの場合があることを ambiguous case といいます．
False
https://www.proprofs.com/discuss/q/592712/you-can-have-decimal-oneths-5gb
(2) $\displaystyle \frac{4+\sqrt{6}}{2}$
3
2)
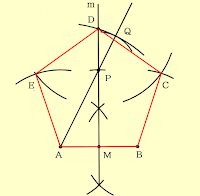
①線分ABの垂直2等分線mを引き、線分ABの中点をMとする。
300π=k×10×30
k=π
A=πab
(b) x=8tan70°=22.0
(c) α=arccos(7/12)=54.3°, β=90-54.3=35.7°
a) $p(x)=r(x)-c(x)=4 \sqrt x-2x^2$
$=4x^{\frac{1}{2}}-2x^2$
Q1.
a. W(2)=(0,1), W(3)=(0,0), W(4)=(1,0), W(5)=(1,1)
b. 単位正方形を1周，2周，…と包んでいくので周期関数になる．周期は4．
c. 通る点が周期的になるので，その座標も周期的になる．
d. nを整数（n∈$\mathbb{ Z }$），[ ]をガウス記号とすると，
4n≤t≤4n+1のとき，W(t)=(1, t-[t])
4n+1≤t≤4n+2のとき，W(t)=(1-t+[t], 1)
4n+2≤t≤4n+3のとき，W(t)=(0, 1-t+[t])
4n+3≤t≤4(n+1)のとき，W(t)=(t-[t], 0)
よって，u=c(t)とu=s(t)のグラフは，
点(1,0)を起点として単位円を反時計回りに包む円弧の長さがtのときの先端の座標を(cost, sint)と定義する．
(1) The problem can be restated as an equation of the form 300x+210y=d, where x is the number of pigs, y is the number of goats, and d is the positive debt. The problem asks us to find the lowest d possible. x and y must be integers, which makes the equation a Diophantine equation. The Euclidean algorithm tells us that there are integer solutions to the Diophantine equation ax+by=d, where d is the greatest common divisor of x and y, and no solutions for any smaller d. Therefore, the answer is the greatest common divisor of 300 and 210, which is 30, (C).
(2) 95x+97y=4238 ⇔ 95x+97(y-43)=67 ⇔ 95p+97q=1 ⇔ 95･48+97･(-47)=1 ⇔ 95･3216+97･(-3149)=67 ⇔ x=3216+97k=15+97(33+k) ⇔ Donut=15
Jack must pay 15･0.95=14.25, and Jill must pay 29･0.97=28.13. The total to pay is 42.38, which accords with the total bill.
（途中，$\dfrac{h}{x}$を$d$と置き換えています）$$\begin{align}(\ln x)' &= \lim_{h\to 0}\frac{\ln(x+h)-\ln x}{h} \\ &= \lim_{h\to 0}\dfrac{1}{h}\ln \left( \dfrac{x+h}{x}\right) \\ &= \lim_{h\to 0}\ln \left( 1+\dfrac{h}{x}\right)^\dfrac{1}{h}\\ &= \lim_{d\to 0}\ln \left( 1+d \right)^\dfrac{1}{dx}\\&= \lim_{d\to 0}\dfrac{1}{x}\ln \left( 1+d\right)^\dfrac{1}{d} \\ &=\dfrac{1}{x}\ln e
\\ &= \dfrac{1}{x}\end{align}$$
[Midsegment Theorem 解答]
Cantor set は1回の操作につき，長さ1/3の相似図形が2つ残るので，そのfractal dimensionは，
Koch curve は1回の操作につき，長さ1/3の相似図形が4つ残るので，そのfractal dimensionは，
このように実数解が2つ，虚数解が2つありますが，最も簡単な値（x=5）のときだけ周長まで計算してみましょう．x=5のとき，長い方の対角線の長さの1/2は2x-2=8です．このとき対角線のなす角もαなので，$$\sin(\pi-\alpha)=\frac{3}{5} \space\space\space\space \cos(\pi-\alpha)=-\frac{4}{5}$$となって，余弦定理より，$$b^2=5^2+8^2-2\cdot5\cdot8\cdot \cos(\pi-\alpha)=153$$$$b=\sqrt{153}=3\sqrt{17}$$従って，x =5のときのrhomboidの周長のexact valueは$$2a+2b=10+6\sqrt{17}$$となり，近似値は約34.7となります．
b=8, c=7, m=6, n=5, a=11なので，Cevian ADの長さは式(4)より
$$\sqrt{\frac{299}{11}}\approx5.21$$
$B_2=180-75.8=104.2$
$B=75.8°$ or $104.2°$
このように2通りの場合があることを ambiguous case といいます．
[At Most 解答]
$\displaystyle q=-\frac{1}{6}x^3+\frac{13}{6}x+1$
$\displaystyle q=-\frac{1}{6}x^3+\frac{13}{6}x+1$
[Scale Factor 解答]
a) 12:5:13
b) 63:67
a) 12:5:13
b) 63:67
[HCF 解答]
240 = 2 x 2 x 2 x 2 x 3 x 5
924 = 2 x 2 x 3 x 7 x 11
The HCF is 2 x 2 x 3 = 12
[Oneth 解答]
False
https://www.proprofs.com/discuss/q/592712/you-can-have-decimal-oneths-5gb
[Riemann Sum 解答]
$f(t)=-t^3+4$とすると，$\displaystyle \frac{2}{5} \left( f(-1)+f\left(-\frac{3}{5}\right)+f\left(-\frac{1}{5}\right)+f\left(\frac{1}{5}\right)+f\left(\frac{3}{5}\right) \right)=8.4$
（定積分の正確な値は8になります）
[PEMDAS / BODMAS 解答]
（WolframAlphaやグラフ電卓TI84などでは）$12/3x-3+4=12÷3×2-3+4$
$=4×2-3+4=8-3+4=5+4=9$
（日本の学習指導要領中学数学では）$12/3x-3+4=12÷(3×2)-3+4$
$=12÷6-3+4=2-3+4=-1+4=3$
参照 WikipediA "Order of operations"
[Radicals and Surds 解答]
(1) b(2) $\displaystyle \frac{4+\sqrt{6}}{2}$
[Continued Square Roots 解答]
3
[Ferris Wheel 解答]
(a) a=13, b=12, c=π/30, d=15 (b) 24.9 m
(a) a=13, b=12, c=π/30, d=15 (b) 24.9 m
[Straightedge and Compass Construction 解答]
1)
②直線m上（線分ABの上側）に、AB=MPとなる点Pをとる。
③APの延長上に、AM=PQとなる点Qをとる。
④Aを中心としてAQを半径とする円と、直線mとの交点（線分ABの上側）をDとする。
⑤Dを中心としてABを半径とする円と、Aを中心として同じ半径の円の交点（直線mの左側）をEとする。
⑥Dを中心としてABを半径とする円と、Bを中心として同じ半径の円の交点（直線mの右側）をCとする。
⑦B, C, D, E, Aを結ぶ。
[Direct Variation 解答]
Let A=kab300π=k×10×30
k=π
A=πab
[Pronumeral 解答]
(a) θ=arctan(15/5)=71.6°(b) x=8tan70°=22.0
(c) α=arccos(7/12)=54.3°, β=90-54.3=35.7°
[Radix Point 解答]
[Fractional Part 解答]
$5^2=25$，$6^2=36$だから，xのinteger partは5と推測できるので，x=5+tとおくと，$x^2+\{ x \}^2=27$より，$(5+t)^2+t^2=27$
これを解いて，$t=\displaystyle \frac{-5+\sqrt{29}}{2}$
$5^2=25$，$6^2=36$だから，xのinteger partは5と推測できるので，x=5+tとおくと，$x^2+\{ x \}^2=27$より，$(5+t)^2+t^2=27$
これを解いて，$t=\displaystyle \frac{-5+\sqrt{29}}{2}$
よって，$x=5+t=\displaystyle \frac{5+\sqrt{29}}{2}$
[Inside and Outside Function 解答]
仮定 $g∘h(x)=2x^2+3x\hspace{ 203pt } (1)$
仮定 $h∘g(x)=x^2+2x-2\hspace{ 190pt } (2)$
式(2)に$x=-2$を代入すると，$h∘g(-2)=-2\hspace{ 130pt } (3)$
ここで$g(-2)=t$とおくと式(3)は $h(t)=-2$ だから， $g∘h(t)=g(-2)\hspace{33pt } (4)$
式(1)より $g∘h(t)=2t^2+3t$なので，式(4)は $2t^2+3t=t$
これを解いて，$t=0, -1$ すなわち $g(-2)=0, -1$
選択肢の中からは，(b) $-1$ が正解．
[Plan and Elevation 解答]
 |
| (a) |
 |
| (b) |
 |
| (c) |
[Lagrange's Notation, Leibniz's Notation 解答]
a) $p(x)=r(x)-c(x)=4 \sqrt x-2x^2$$=4x^{\frac{1}{2}}-2x^2$
b) $\displaystyle \frac{dp}{dx}=2x^{-\frac{1}{2}}-4x, \ \frac{d^2p}{dx^2}=-x^{-\frac{3}{2}}-4$
c) $\displaystyle \frac{dp}{dx}=0 \Leftrightarrow x=2^{-\frac{2}{3}}=0.6299...$
maximum 0.630 thousands (or 630)
[Wrapping Function 解答]
Q1.
a. W(2)=(0,1), W(3)=(0,0), W(4)=(1,0), W(5)=(1,1)
b. 単位正方形を1周，2周，…と包んでいくので周期関数になる．周期は4．
c. 通る点が周期的になるので，その座標も周期的になる．
d. nを整数（n∈$\mathbb{ Z }$），[ ]をガウス記号とすると，
4n≤t≤4n+1のとき，W(t)=(1, t-[t])
4n+1≤t≤4n+2のとき，W(t)=(1-t+[t], 1)
4n+2≤t≤4n+3のとき，W(t)=(0, 1-t+[t])
4n+3≤t≤4(n+1)のとき，W(t)=(t-[t], 0)
よって，u=c(t)とu=s(t)のグラフは，
u=c(t) u=s(t)
Q2.点(1,0)を起点として単位円を反時計回りに包む円弧の長さがtのときの先端の座標を(cost, sint)と定義する．
[Diophantine Equations 解答]
(1) The problem can be restated as an equation of the form 300x+210y=d, where x is the number of pigs, y is the number of goats, and d is the positive debt. The problem asks us to find the lowest d possible. x and y must be integers, which makes the equation a Diophantine equation. The Euclidean algorithm tells us that there are integer solutions to the Diophantine equation ax+by=d, where d is the greatest common divisor of x and y, and no solutions for any smaller d. Therefore, the answer is the greatest common divisor of 300 and 210, which is 30, (C).
(2) 95x+97y=4238 ⇔ 95x+97(y-43)=67 ⇔ 95p+97q=1 ⇔ 95･48+97･(-47)=1 ⇔ 95･3216+97･(-3149)=67 ⇔ x=3216+97k=15+97(33+k) ⇔ Donut=15
Jack must pay 15･0.95=14.25, and Jill must pay 29･0.97=28.13. The total to pay is 42.38, which accords with the total bill.
[First Principles 解答]
（途中，$\dfrac{h}{x}$を$d$と置き換えています）$$\begin{align}(\ln x)' &= \lim_{h\to 0}\frac{\ln(x+h)-\ln x}{h} \\ &= \lim_{h\to 0}\dfrac{1}{h}\ln \left( \dfrac{x+h}{x}\right) \\ &= \lim_{h\to 0}\ln \left( 1+\dfrac{h}{x}\right)^\dfrac{1}{h}\\ &= \lim_{d\to 0}\ln \left( 1+d \right)^\dfrac{1}{dx}\\&= \lim_{d\to 0}\dfrac{1}{x}\ln \left( 1+d\right)^\dfrac{1}{d} \\ &=\dfrac{1}{x}\ln e
\\ &= \dfrac{1}{x}\end{align}$$
[Explicit Formula 解答]
上の$C_n=2^n$は$C_n=2^{n-1}$に，$2^6=32$は$2^5=32$に訂正してください．
正解は$2^{n-1}$ではなく，$\displaystyle \frac{1}{24}(n^4-6n^3+23n^2-18n+24)$になります．その理由はMethod of Differencesで見てください．
(Moser's Circle Problem)
a) 18.6
b) 30.2
[Bearings 解答]
a) Boat A: (x1(t), y1(t))=(2, 4)+t(1, -3) より x1(t)=2+t, y1(t)=4–3t, t ≥0
1. $\displaystyle \int{x^2\log x}dx=\frac{1}{9}x^3(3\log x-1)+C$
[Significant Figures 解答]
GDCを利用して，V=integral from a to b PI y^2 dx よりa) 18.6
b) 30.2
a) Boat A: (x1(t), y1(t))=(2, 4)+t(1, -3) より x1(t)=2+t, y1(t)=4–3t, t ≥0
b) Boat B: (x2(t), y2(t))=(11, 3)+(t-2)(-1, a) より x2(t)=13–t, y2(t)=3–2a+at, t ≥2
c) 2+t=13–t より t=11/2 だから 2:22:30 pm
d) このとき 4-3t=3–2a+at より a=-31/7 なので 速度ベクトルは v=(-1, -31/7) となり Tan-1(7/31)≈13º だから bearing≈13º west of south (or 193º)
|v|=√(1+a2)=√(1010/49)≈4.54
[Tabular Method 解答]
1. $\displaystyle \int{x^2\log x}dx=\frac{1}{9}x^3(3\log x-1)+C$
2. $\displaystyle \int{x^6e^x}dx=e^x (x^6-6x^5+30x^4-120x^3+360x^2-720x+720)+C$
3. $\displaystyle \int{e^{-x}\sin x}dx=-\frac{1}{2}e^{-x}(\sin x+\cos x)+C$
[R-Alpha Method 解答]
左辺を変形すると，
$\sqrt{52}\cos(x–33.7°)=3$
これを解いて，
x=99°，328°
ところが，右図のようにグラフ電卓を使うと，左辺のグラフと右辺のグラフの交点を求めれば，式変形しなくても解けます．
 |
| Cantor set |
log32=0.6309297536...≈0.63
 |
| Koch curve |
log34=1.261859507...≈1.26
$A(3)=2340.00\times e^{0.031\times 3}\approx2568.06$
$R=\log_{10}\frac{4I}{I_0}=\log_{10}4+\log_{10}\frac{I}{I_0}\approx0.6+8.3=8.9$
$12x^2-61x-16$のAC=-192, B=-61なので，積が-192で和が-61になる2数は3と-64．よって，$12x^2+3x-64x-16=3x(4x+1)-16(4x+1)=(3x-16)(4x+1)$
$$0<\frac{n^n}{(2n)!}=\frac{n^n}{2n(2n-1)\cdot\cdot\cdot(n+1)n!}<\frac{n^n}{n^n\cdot n!}=\frac{1}{n!}$$右辺の極限値は0なので, $$\lim\limits_{n\to\infty}\frac{n^n}{(2n)!}=0$$
(1) 余弦定理より$$\sqrt{28}=2\sqrt{7}\approx5.29$$(2) A=53°18'=53.3°，B=48°36'=48.6°より，C=180-53.3-48.6=78.1°で正弦定理より$$\frac{\sin{C}}{c}=\frac{\sin{B}}{b}\Leftrightarrow\frac{\sin{78.1^\circ}}{652}=\frac{\sin{48.6^\circ}}{b}\Leftrightarrow b=\frac{652\cdot\sin{48.6^\circ}}{\sin{78.1^\circ}}=499.8$$$$w=b\sin{53.3^\circ}=400.7\approx401$$
短い方の対角線の長さを2xとし，長い方の対角線と底辺とのなす角をαとします．平行四辺形の面積が48で底辺が5なので，高さは48/5となります．なのでsinの定義より$$\sin\alpha=\frac{\frac{24}{5}}{2x-2} $$一方，余弦定理より，$$\cos\alpha=\frac{5^2+(2x-2)^2-x^2}{2\cdot5\cdot(2x-2)}$$これらを$$\sin^2x+\cos^2x=1$$に代入して整理すると， \[9x^4-48x^3-162x^2+336x+2745=0 \] となるので，この4次方程式を解かなければなりません．手計算では困難なのでグラフ電卓を使って，左辺の4次関数の零点（x軸との交点）を求めましょう．
[Compound Interest 解答]
$A(3)=2340.00\times e^{0.031\times 3}\approx2568.06$
[Magnitude 解答]
$R=\log_{10}\frac{4I}{I_0}=\log_{10}4+\log_{10}\frac{I}{I_0}\approx0.6+8.3=8.9$
[Crisscross Method 解答]
$12x^2-61x-16$のAC=-192, B=-61なので，積が-192で和が-61になる2数は3と-64．よって，$12x^2+3x-64x-16=3x(4x+1)-16(4x+1)=(3x-16)(4x+1)$
[Squeeze Theorem 解答]
$$0<\frac{n^n}{(2n)!}=\frac{n^n}{2n(2n-1)\cdot\cdot\cdot(n+1)n!}<\frac{n^n}{n^n\cdot n!}=\frac{1}{n!}$$右辺の極限値は0なので, $$\lim\limits_{n\to\infty}\frac{n^n}{(2n)!}=0$$
[Oblique Triangle 解答]
(1) 余弦定理より$$\sqrt{28}=2\sqrt{7}\approx5.29$$(2) A=53°18'=53.3°，B=48°36'=48.6°より，C=180-53.3-48.6=78.1°で正弦定理より$$\frac{\sin{C}}{c}=\frac{\sin{B}}{b}\Leftrightarrow\frac{\sin{78.1^\circ}}{652}=\frac{\sin{48.6^\circ}}{b}\Leftrightarrow b=\frac{652\cdot\sin{48.6^\circ}}{\sin{78.1^\circ}}=499.8$$$$w=b\sin{53.3^\circ}=400.7\approx401$$
[Rhomboid 解答]
よって，x=5 および x=5.6828668…ですが，”exact value”（近似値でなく根号やπなどを用いた値）で答えなければならないので， WolframAlphaに計算してもらうと，2つの実数解と2つの虚数解になり，実数解のひとつであるx=5.68…の方のexact valueは大変な値になりました．下図の上から2つ目のxです．
[Cevian 解答]
b=8, c=7, m=6, n=5, a=11なので，Cevian ADの長さは式(4)より
$$\sqrt{\frac{299}{11}}\approx5.21$$
